Computational modeling of spinal circuits controlling limb coordination and gaits in quadrupeds
- Drexel University College of Medicine, United States
- Université de Sherbrooke, Canada
Figures
Figure 1
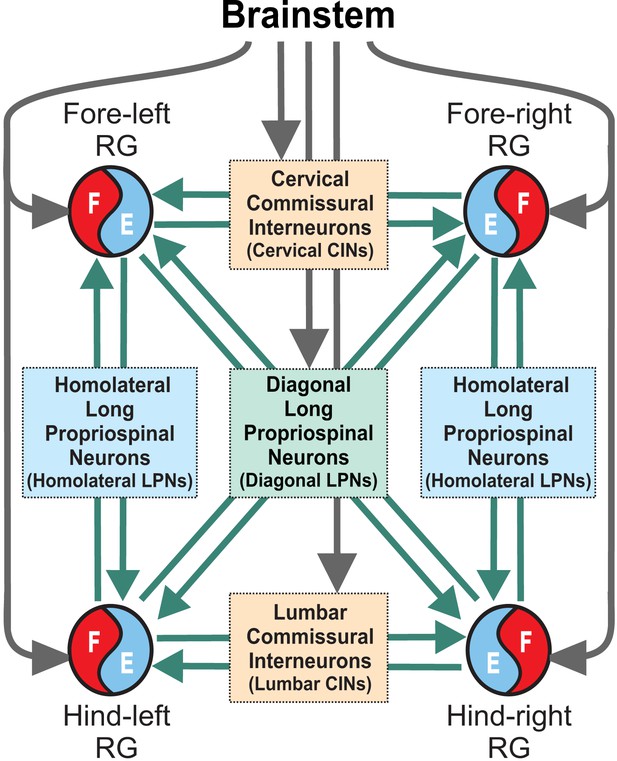
Model concept.
Each limb is controlled by a separate rhythm generator (RG). The local commissural neurons (CINs) as well as homolaterally and diagonally projecting (descending and ascending) long propriospinal neurons (LPNs) couple the four RGs. Brainstem drive acts on the RGs to control locomotor speed and on CINs and diagonal LPNs to control gaits.
Figure 2
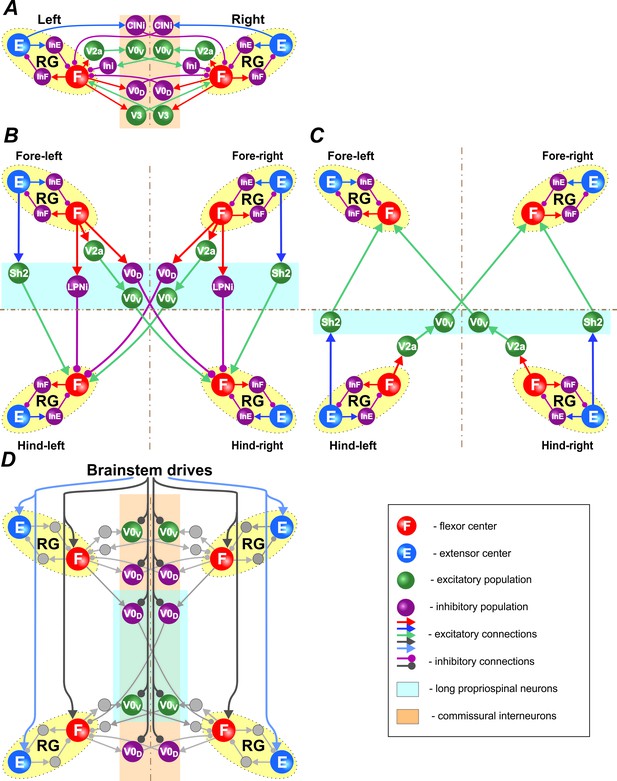
Connections within the spinal cord.
(A) Connections between left and right rhythm generators (RG) within each girdle. (B) Connections from the fore to hind RGs via descending (cervical-to-lumbar) long propriospinal neurons (LPNs). (C) Connections from the hind to fore RGs via ascending (lumbar-to-cervical) LPNs. (D) Brainstem drive connections to the extensor and flexor centers, commissural interneurons, and LPNs. Spheres represent neural populations. Excitatory and inhibitory connections are marked by arrowheads and circles, respectively.
Figure 3
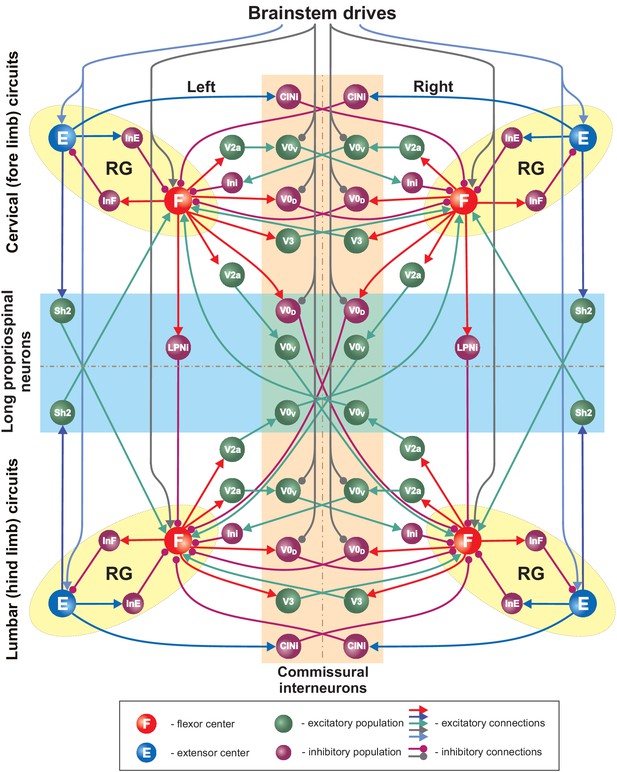
Full model schematic.
Spheres represent neural populations and lines synaptic connections. Excitatory and inhibitory connections are marked by arrowheads and circles, respectively. RG, rhythm generator.
Figure 4
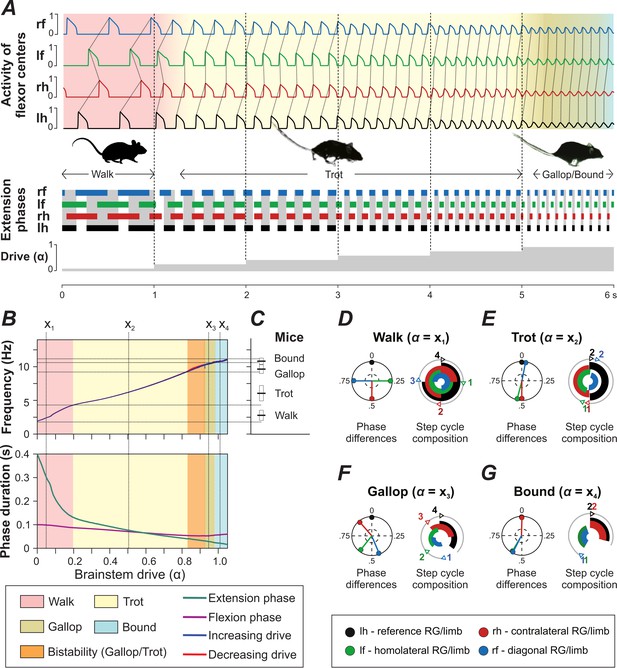
Model performance.
(A) Gait changes during 6 s of simulation with increase of brainstem drive (parameter ) every second (shown at the bottom). Gait changes were subject to a brief transitional period (shown as transitional colors). (B) Dependency of frequency and phase durations on . Vertical dashed lines (labeled as ) represent selected values of related to different gaits. Note that the blue line almost completely covers the red one (indicating increase and decrease of , respectively) in the top diagram. (C) Frequency ranges in which gaits are observed in mice (created from data from Figure 1E of Bellardita and Kiehn, 2015). (D–G) Phase differences and step cycle compositions of four characteristic gaits produced by the model that correspond to the -values () in B.
Figure 5
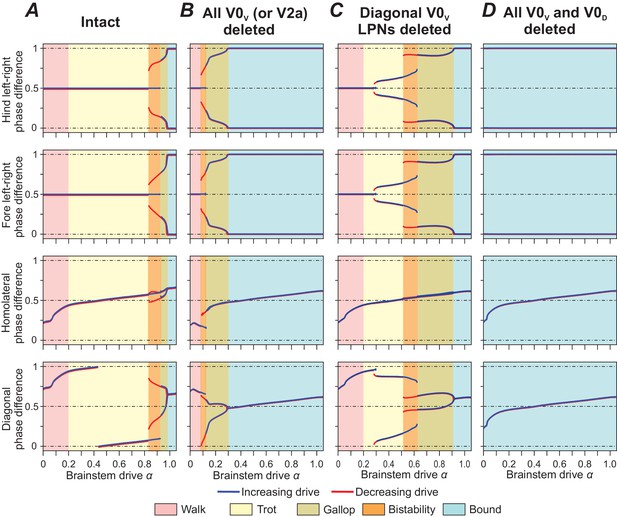
Bifurcation diagrams of the intact model (A), after removal of all V0V neurons (B), of only V0V LPNs (C), and of all V0V and V0D neurons (D).
Normalized phase differences of 0.5 correspond to alternation, whereas phase differences of 0 or 1 correspond to synchronization. Blue and red lines indicate the stable phase differences with stepwise increase and decrease of the bifurcation parameter , respectively. Colored areas indicate the expressed gait.
Figure 6
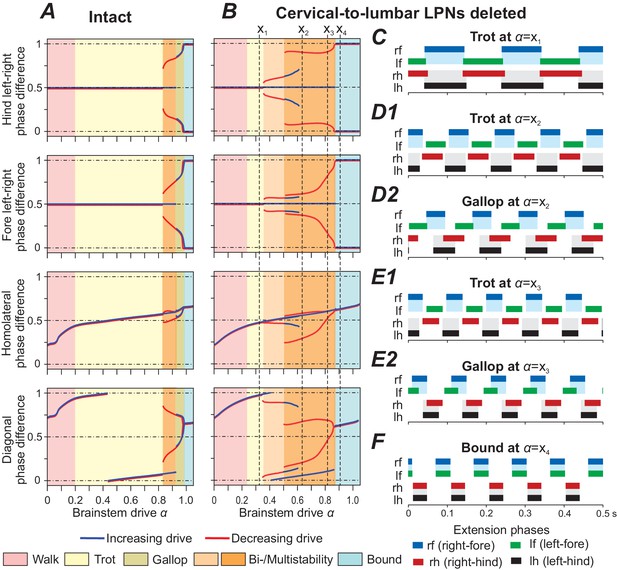
Model performance after deletion of all cervical-to-lumbar long propriospinal neurons (LPNs).
Bifurcation diagrams, indicating the stable phase differences depending on the bifurcation parameter for the intact model (A) and after deletion of all cervical-to-lumbar LPNs (B). (C–F) Extension phases of stable gaits (identified by vertical dashed lines in B labeled as ). At two different gaits were stable simultaneously (depicted in D1, D2 and E1, E2, respectively).
Figure 7
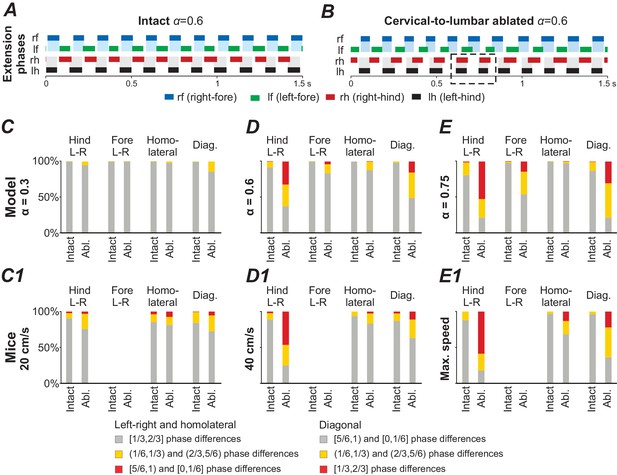
Model performance under application of noise before (intact) and after ablation of cervical-to-lumbar long projecting propriospinal neurons (LPNs).
(A,B) Exemplary extension phases of the intact model (A) and after removal of cervical-to-lumbar LPNs (B). The dashed box in B indicates synchronization of hind RGs that transiently occurred after removal of cervical-to-lumbar LPNs. (C–E,C1–E1) Phase differences categorized into three equally sized bins (gray: appropriate for trot, yellow: quarter phase difference off, red: antiphase) before and after ablation of cervical-to-lumbar LPNs for 1000 s simulation in the model (C–E) and for experimental data pooled across animals (C1–E1) at three different speeds and -values. C1–E1 were created from data extracted from Figure 5B,C and S5A,D of Ruder et al. (2016).
Figure 8
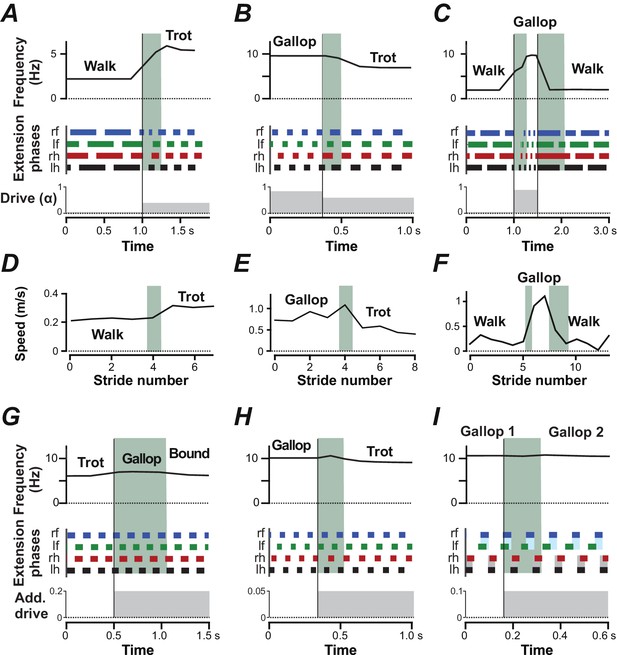
Gait transitions with changes of brainstem drive (A–C) and independent of brainstem drive (G–I).
(A–C) Instantaneous frequency, extension phases before and after parameter was abruptly changed (bottom trace). Black vertical lines indicate the time when was changed and the shaded green areas indicate the transitions periods. (A) Transition from walk to trot when was changed from 0.02 to 0.4. (B) Transition from gallop to trot when was changed from 0.85 to 0.6. (C) Transition from walk to gallop and back to walk when was changed from 0.02 to 0.9 and to 0.02. (D–F) Experimentally observed instantaneous speed during gait changes in mice corresponding to A–C (created from data extracted from Figure 3A–C of Bellardita and Kiehn 2015). (G–I) Instantaneous frequency and extension phases during gait changes caused by additional drives to CINs and LPNs. Bottom trace indicates time course of additional drives. (G) Additional inhibitory drive to all V0V CINs and LPNs (, ) at and caused a transition from trot to bound. During the transitional period a gallop occurred. (H) An additional excitatory drive to local V0V CINs (, ) at caused a transition from gallop to trot. (I) Additional excitatory drive to cervical, local V0V CINs (, ) at caused the left-right phase difference of the fore RGs to change from almost synchronized to a quarter-phase lag during gallop.
Figure 9
Schematic representation of control of limb coordination and gait by different local commissural interneurons (CINs) and long propriospinal neurons (LPNs).
(A) Circuits controlling four limbs. Each limb is controlled by its own rhythm generator (RG). Local CINs and homolateral and diagonal LPNs couple the RGs and coordinate their activities. Supraspinal signals and sensory feedbacks (directly or also indirectly through dorsal horn interneurons; not shown) provide inputs to the RGs, CINs and LPNs. Inputs to the RGs affect the locomotor frequency (speed), while inputs to CINs and LPNs affect interlimb coordination and gait expression. (B) A more detailed representation of local CINs and LPNs that are located in one cord section (here left cervical) and integrate the corresponding intraspinal and supraspinal inputs and sensory information (from the fore left limb) to mediate the effect of this limb’s activity on spinal circuits controlling the other three limbs.
Tables
Table 1
Connection weights.
https://doi.org/10.7554/eLife.31050.011| Source | Target () |
|---|---|
| Within girdle and side of the cord | |
| RG-F | i-Ini-F (0.40), i-V0D (0.70), i-V2a (1.00), i-V3 (0.35), i-V2a-diag (0.50) |
| f-RG-F | i-Ini-Hom (0.70), i-V0D-diag (0.50) |
| RG-E | i-Ini-E (0.40), i-CINi (0.40), i-Sh2-Hom (0.50) |
| Ini-F | i-RG-E (–1.00) |
| Ini-E | i-RG-F (–0.08) |
| V2a | i-V0V (1.00) |
| V2a-diag | i-V0V-diag (0.90) |
| IniV0V | i-RG-F (–0.07) |
| Between left and right homologous circuits | |
| V0D | c-RG-F (–0.07) |
| V0V | c-IniV0V (0.60) |
| V3 | c-RG-F (0.03) |
| CINi | c-RG-F (–0.03) |
| Between fore and hind homolateral circuits | |
| f-Ini-Hom | h-RG-F (–0.01) |
| f-Sh2-Hom | h-RG-F (0.01) |
| h-Sh2-Hom | f-RG-F (0.125) |
| Between diagonal circuits | |
| f-V0D-diag | dh-RG-F (–0.075) |
| f-V0V-diag | dh-RG-F (0.02) |
| h-V0V-diag | df-RG-F (0.065) |
-
i-, ipsilateral; c-, contralateral; f-, fore; h-, hind; d, diagonal. CINi, inhibitory commissural interneurons. Ini, regular inhibitory interneurons. RG-F, flexor center; RG-E, extensor center.
Table 2
Operational definition of gaits.
https://doi.org/10.7554/eLife.31050.012| Normalized phase differences | |||
|---|---|---|---|
| Gait | Left-right hind | Homolateral | Diagonal |
| Walk* | [0.25,0.75] | [0.1,0.4) and (0.6,0.9] | (0.1,0.4] and [0.6,0.9) |
| Trot | [0.25,0.75] | [0.25,0.75] | [0.0,0.1] and [0.9,1.0) |
| Gallop | (0.025,0.25] and [0.75,0.975) | [0.25,0.75] | [0.25,0.75] |
| Bound | [0.0,0.025] and [0.975,1.0) | [0.25,0.75] | [0.25,0.75] |
-
*Classification of walk additionally required longer extension than flexion phase durations.
Additional files
-
Transparent reporting form
- https://doi.org/10.7554/eLife.31050.013
Download links
A two-part list of links to download the article, or parts of the article, in various formats.
Downloads (link to download the article as PDF)
Open citations (links to open the citations from this article in various online reference manager services)
Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)
Computational modeling of spinal circuits controlling limb coordination and gaits in quadrupeds
eLife 6:e31050.
https://doi.org/10.7554/eLife.31050
