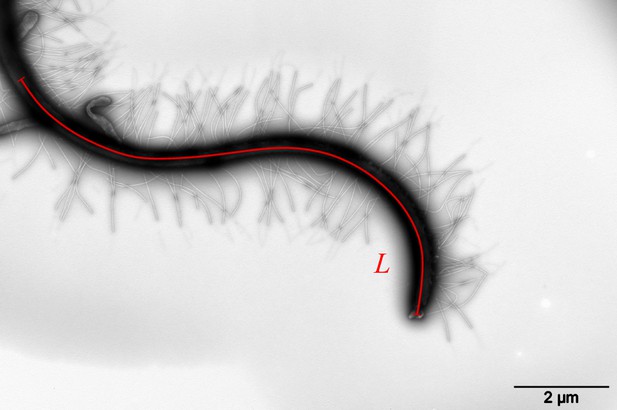
Coordination of two opposite flagella allows high-speed swimming and active turning of individual zoospores
Figures
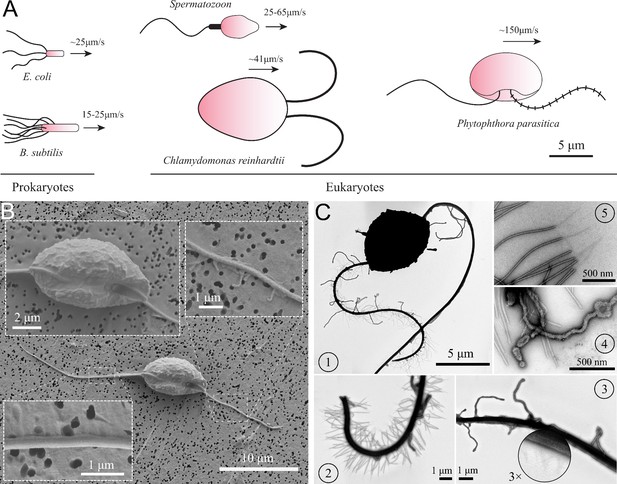
Characteristics of P. parasitica zoospore.
(A) Swimming of zoospores in comparison with different prokaryotic and eukaryotic microswimmers. Black arrows indicate the swimming direction of the swimmers. (B) Scanning electron microscopy images of the zoospore. The insets show the enlarged images of the cell body and the two flagella. (C) Transmission electron microscopy images with negative staining. (1) Image of the zoospore showing the different structures of the two flagella. The anterior has multiple mastigonemes, while the posterior has a smooth straight structure. (2) Close zoom-in image of the anterior flagellum. It is noticed that there are two types of mastigonemes on this flagellum: one with straight tubular shape, the other with non-tubular shape but longer and bigger in size. (3) Close zoom-in image of the posterior flagellum. There are plenty of thin and short hairs wrapping along the flagellum and several non-tubular mastigonemes appearing near the cell body. (4) The non-tubular mastigonemes. (5) The tubular mastigonemes with tiny hairs at the tips.
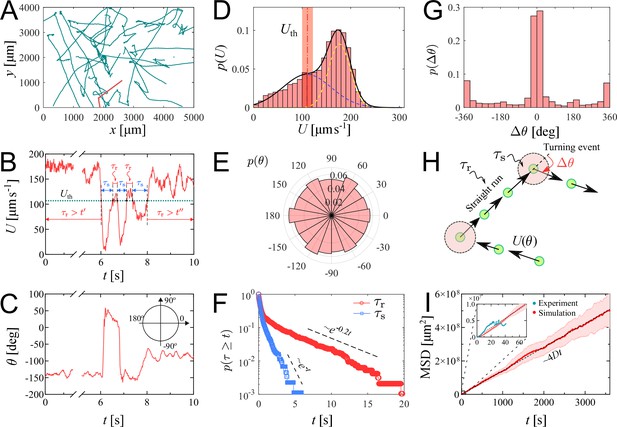
Swimming trajectories of P. parasitica zoospores.
(A) Trajectories of zoospores swimming in water captured from the microscopic assay for 60 s. Sample size . Note: not all trajectories are shown. Each position of the zoospores is captured every . The trajectories are smoothed with moving average (step length ). (B) The progression of speed and (C) moving directions θ over time of a single zoospore extracted from the population in the assay. (D) Distribution of zoospore speed . (E) Polarity distribution of moving direction . (F) Survival curves of the running time and stopping time . (G) Distribution of turning angle , with positive angle values indicating counter-clockwise, and negative as clockwise direction. (H) Schematics showing the strategy of the simulation model of zoospores swimming in water. (I) The estimated mean squared displacement (MSD) over time intervals , constructed from the simulation data. The inset compares the experimental data and simulation of MSD at the experimental time-scale of 60 s. By simulation, at long time scale of 1 h, MSD of zoospores shows a diffusion of Brownian particles with the diffusion coefficient .
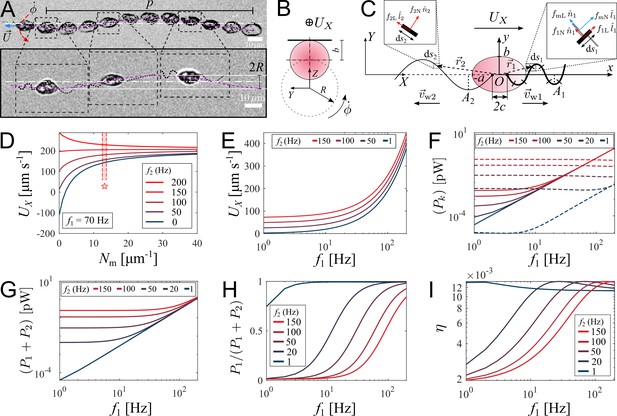
Theoretical model of swimming individual P. parasitica zoospore.
(A) Images of an individual zoospore swimming with two flagella beating in sinuisoidal waveform shapes and its cell body gyrating with rate while moving forward with speed . The combined motion results in a helical swimming trajectory with pitch and radius . (B) Schematics showing the gyration of the cell body. (C) Theoretical model of a zoospore translating in a 2D plane using Resistive Force Theory. (D) The dependence of translational speed on the type-1 mastigoneme density (). The range of with symbol (⋆) indicates the values measured by TEM. (E) The effects of beating frequencies of the two flagella, f1 and f2, on zoospore speed , (F) power consumption of each flagellum , (G) total power consumption of two flagella (), (H) power distributed to the anterior flagellum and (I) propelling efficiency of both flagella η. In these plots, is set at 13 .
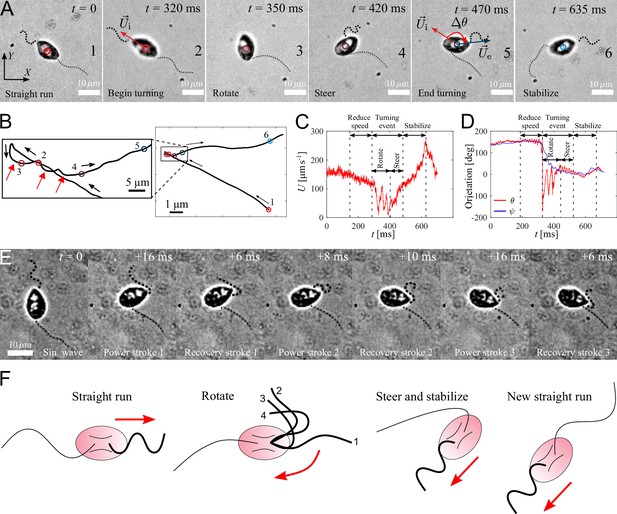
Active turning of individual P. parasitica zoospores in water.
(A) Images of a zoospore changing direction. The two flagella cooperate to help the cell body rotate and steer to a new direction achieving a turning angle . (B) Trajectory of the zoospore during the turning event. Three red arrows represent 3 back and forth stroke-like motions. (C) The speed of the zoospore during the turning event. The turning starts when the speed begins to fluctuate with large magnitude and lower frequency, and lasts for a duration of with a rotation of the cell body followed by steering to the new direction. (D) The moving directions θ and the body orientation ψ of the zoospore during the turning event. (E) Images of the anterior flagellum of a zoospore beats with power and recovery stroke, similar to C. reinhardtii’s in a temporal zoom corresponding to the "Rotate" step of the turning event (but not from the same video as (A)). (F) Schematic to describe the gait of the flagella during a turning event. (1-2) Power stroke 1, (2-3) recovery stroke 1, (3-4) power stroke 2.
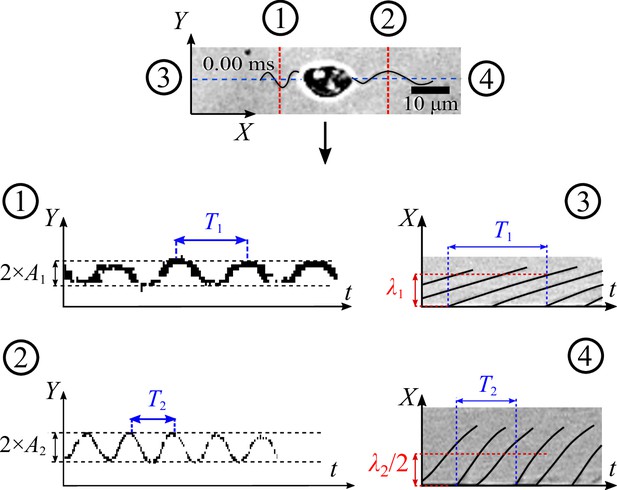
Strategy to apply kymograph to obtain characteristics of beating zoospore flagella.
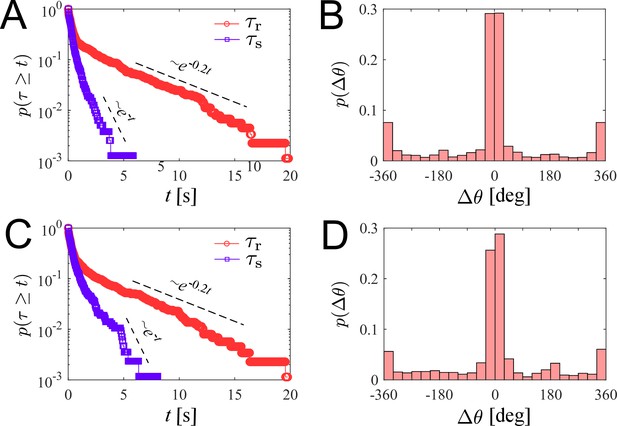
Estimation of , and for different variation of .
(A) , and (B) for (C), and (D) for .
Videos
Zoospores swimming in thin water film.
The video was captured in the microscopic assay, with objectives 4×, 60 fps, duration 60 s. The trajectories of zoospores were tracked by combining TrackMate (in Fiji) and manual tracking.
A zoospore swimming near water/air interface.
The result from our simulation with the strategy described in Figure 2(H).
The parameters of the simulation are extracted from the statistics of the swimming of zoospores, presented in Figure 2(D–G).
An individual zoospore swimming in water.
First, it swims straight with the helical trajectory due to the spontaneous gyration of the cell body, then performs a 180° turning.
A zoospore performing a turning event, which involves reducing speed, body rotation and steering resulting from active beating of anterior flagellum.
The posterior flagellum is immobile during the turning event.
A zoospore during a turning event changes gait of its anterior flagellum from sinuisoidal wave to power and recovery stroke, similar to C. reinhardtii’s.
Tables
Characteristics of helical trajectories of zoospores during straight runs.
| Sample | Pitch () | Radius () | Gyrational speed () |
|---|---|---|---|
| 1 | 122 | 3.250 | 3.64 π |
| 2 | 123 | 4.435 | 4.00 π |
| 3 | 145 | 4.129 | 2.86 π |
| 4 | 164 | 3.390 | 2.5 π |
| 5 | 178 | 4.850 | 2.1 π |
| 6 | 112 | 3.010 | 4.44 π |
| 7 | 120 | 4.300 | 4.44 π |
| 8 | 96 | 4.244 | 5 π |
| 9 | 127 | 4.090 | 3.33 π |
| 10 | 106 | 4.744 | 4 π |
Measurement data of mastigonemes in TEM images.
| Sample | No. of mastigonemes | Flagellum length () | Density () |
|---|---|---|---|
| 1 | 152 | 11.842 | 12.8357 |
| 2 | 168 | 11.597 | 14.4865 |
| 3 | 113 | 8.627 | 13.0984 |
| 4 | 181 | 13.590 | 13.3186 |
| 5 | 198 | 15.195 | 13.0306 |
| 6 | 177 | 13.994 | 12.6483 |
| 7 | 88 | 7.499 | 11.7349 |
Physical parameters of beating flagella of P parasitica zoospores in normal conditions.
| Structures | Parameters | Denotes | Value |
|---|---|---|---|
| Anterior | Amplitude () | A1 | 1.6 |
| flagellum | Wavelength () | 7.5 | |
| Length () | L1 | 23.4 | |
| Beating freq. (Hz) | f1 | 74 | |
| Drag coeff. of filaments | |||
| () | |||
| Drag coeff. of mastigonemes | |||
| () | |||
| Mastigoneme length () | 1.5 | ||
| Mastigoneme density () | 13 | ||
| ratio | 0.21 | ||
| Drag coeff. ratio () | 2.047 | ||
| Posterior | Amplitude () | A2 | 2.9 |
| flagellum | Wavelength () | 15.17 | |
| Beating freq. (Hz) | f2 | 128 | |
| Length () | L2 | 25.8 | |
| Drag coefficients | |||
| () | |||
| ratio | 0.19 | ||
| Drag coeff. ratio () | 0.3534 |